N by N chess games
SEQUENCE OF (2k+1) x (2k+1) CHESS GAMES
by A. Missoum
1) For k=1 we have the (2x1+1) x(2x1+1) chess game, or the 3x3 chess game. I fact, it is simply a 3x3 Merel game, where two players oppose each other. Each one has three pawns, and the winner is the first who can align horizontally, vertically or diagonally its pawns on the board (see board 1.)

2) For k=2, we have the (2x2+1) x (2x2+1) chess game, or the 5x5 chess game. It is preferable to call it a checker game if we simply use the pawns. See Board(2).

The pawns move and captures as those of the checker game. The 5x5 chess game can also be considered as a 5x5 Merel game, where the winner is one who first align his five pawns horizontally, vertically or diagonally on the squares of the board. We can also conider the 5x5 chess game as follows: The initial arrangement of piece is as in board(3).

Each player has 2 pawns, 1 Firzan which moves and captures like the Firzan(queen) of the Shatranj game (i.e., it moves and captures one square diagonally), 1 King which moves and captures like the King of the 8x8 chess game, and 1 Knight which moves 1 square vertically and one horizonally and vice-versa. The Pawn moves and captures like the Pawn of the chess game. When promoted it can be a Knight, a Firzan or a Pawn.
3)For k=3, we have the (2x3 +1)x (2x3+1) chess game, or the
7x7 chess game. The initial arrangement of pieces(pawns) is
as in board(4). Two players oppose each other, one has black
pieces, and the other has white pieces. Each player has 2 Rooks,
2 knights, 2 Bishops, 1 King and 7 Pawns.

The pawns, the Knight, the Bishop, the Rook and the King move and capture like those of the 8x8 chess game. The prise en passant is still valid. The two Bishops in both side of the King compensate for the lack of the Queen in this game. The castling is still valid that is: the King on d1 moves to f1 and the Rook on g1 moves to e1, or the King on d1 moves to b1 and the Rook on a1 moves to c1. The promoted pawn can be any of the pieces, or a pawn, except for the King. The game ends with the mate of the King of one side.
4) For k=4, we have the (2x4+1) x(2x4+1) chess game, or the 9x9 chess game. The initial arrangement of pieces (pawns) is as in the board(5). Two players oppose each other. One has white pieces, the other has black pieces. Each player has 2 Rooks, 2 Knights, 2 Bishops, 2 Queens, 1 King and 9 pawns. The pieces (Knight, Rook, Queen, King and pawn) move and capture like those of the 8x8 chess game. The introduction of the two queens in both sides of the King fills the remaining two squares d1 and f1, resp d8 and f8) in both sides of the King. In this game, there is no need to replace the Queen by another piece with specific move and capture (for exemple an elephant, a camel, or something else). The Bishop moves and captures only two squares diagonally. In fact, if the Bishop moves and captures like the Bishop of the 8x8 chess, the game became more faster than the 8x8 chess game and therefore less interesting, even it presents some symmetry which is unnecessary. Apparently, the 8x8 chess is does not look a a symmetrical chess game, however, it is devised in a way that the initial arrangement of pieces (pawns) on the chess board reflects the play between a player and its mirror image.
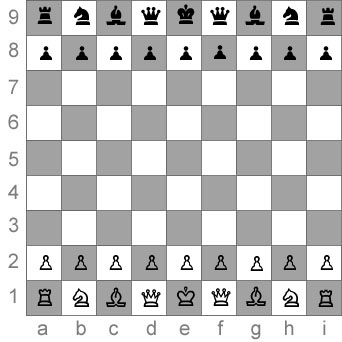
The castling is as follows: King on f1 moves to i1, and Rook on j1 moves to h1, or King on f1 move to b1 and Rook on a1 moves to c1.=20
A promoted pawn can be any of the pieces, or a pawn except for the King. The prise en passant is still valid. At the satrt of the game, the pawn can move two squres at a time.
5) For k= 5, we have the (2x5+1) x (2x5+1) chess game, or the 11 x 11 chess game. Two players oppose each other, one has white pieces, the other has black pieces. Each player has 2 Rooks, 4 knights, 4 Bishops, 1 king and 11 pawns. The initial arrangement of pieces(pawns) is as in board(6). The pieces Rook, Bishop, and pawn move and capture like those of the 8x8 chess game. However, at the start of the game, the pawn can move three, two, or one square at a time. The Knight moves and captures like the Knight of the 8x8 chess game.
In this game, there is no Queen, and this is compensated by the introduction of two bishops and two knights in both sides of the King. Also the King has the option of moving one square or two squares at a time during the game. The castling is as follows:
Small castling: King on f1 moves to i1, and Rook on k1 moves to h1.
Great castling: King on f1 moves to c1, and Rook on a1 moves to d1.
R | N | N | B | B | K | B | B | N | N | R |
P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P |
R | N | N | B | B | K | B | B | N | N | R |
BOARD(6)
6) For k=6, we have the (2x6+1) x (2x6+1) chess game, or the 13 x 13 chess game. Two players oppose each other. One has white pieces, the other has black pieces. Each player has 2 Rooks, 4 Knights, 4 Bishops, two Queens, 1 King and 13 pawns. The initial arrangement of pieces(Pawns) is as in board(7). The Pawns, the Knight, the Queen, and the Rook move and capture like those of the 8x8 chess game. The Bishop is limited to move only a maximum of four squares diagonally.
This will make the game more interesting and less fast as the 8x8 chess game.
At the start of the game, the Pawn can move one, two, three or four squares at a time. The prise en passant is still valid in this game. The promoted pawn can be any of the pieces except for the King. The King has the option of moving one, two or three squares at a time. The castling is as follows :
The great castling: King on g1 moves to c1, and Rook on a1 moves to d1.
The Small castling: King on g1 moves k1 and the Rook on m1 moves to j1.
R | N | N | B | B | Q | K | Q | B | B | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | B | B | Q | K | Q | B | B | N | N | R |
BOARD(7)
7) For k=7, we have the (2x7+1) x (2x7+1) chess game, or the 15 x 15 chess game. Two players oppose each other. One has white pieces, the other has black pieces. Each player has 2 Rooks, 6 Knights, 6 Bishops, two Queens, 1 King, and 15 pawns. The initial arrangement of pieces (pawns) is as in board(8). The Rook, Bishop, Queen move and capture like those of the 8x8 chess game. The knight has the option of moving 3 squares vertically and three squares horizontally and vice-versa, or like the Knight of the 8x8 chess. The King can either move one, or two squares at a time. He moves horizontally, vertically or diagonally. The castling is as follows:
Small Castling: King on h1 moves to m1, and Rook on o1 moves to l1.
Great castling: King on h1 moves to c1 and Rook on a1 moves to
d1.
At the start of the game, the pawn has the option of moving one, two, three or four squares at a time. Then he can only move one square at a time.
R | N | N | N | B | B | B | K | B | B | B | N | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | N | B | B | B | K | B | B | B | N | N | N | R |
BOARD(8)
The lack of the Queen in this game is compensated by the presence of the 6Knights and 6 Bishops in both side of the King.
8) For k=8, we have the (2x8 + 1) x (2 x8 +1) chess game, or the 17 x17 chess game. Two players oppose each other. One has white pieces, the other has black pieces. Each player has 2 Rooks, 6 Knights, 6 Bishops, 2 Queens, 1 King and 17 Pawns. The initial arrangement of piece(pawns) is as in board (9). The Rook, the Queen move and capture as those of the 8x8 chess game. The Bishop can only move a maximum of 5 squares in diagonal. The King can move one, two, three or four squares at a time. At the start of the game, the pawn has the option of moving one two, three, four or five squares at a time The prise en passant is still valid in this game. The knight can either move like the knight of the 13x13 chess, or 15x15 chess.
The castling is as follows:
Small castling: King on i1 moves to n1, rook on q1 moves to m1
Great castling: King on i1 moves to d1, Rook on a1 moves to e1
R | N | N | N | B | B | B | Q | K | Q | B | B | B | N | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | N | B | B | B | Q | K | Q | B | B | B | N | N | N | R |
BOARD(8)
The 17 x17 chess game can be also played as a four handed 8x8 chess game, see board (10).
FOUR HANDED 8x8 CHESS GAME
R | N | B | Q | K | B | N | R | P | R | |||||||
P | P | P | P | P | P | P | P | P | N | |||||||
P | B | |||||||||||||||
P | Q | |||||||||||||||
P | K | |||||||||||||||
P | B | |||||||||||||||
P | N | |||||||||||||||
P | R | |||||||||||||||
R | P | |||||||||||||||
N | P | |||||||||||||||
B | P | |||||||||||||||
K | P | |||||||||||||||
Q | P | |||||||||||||||
B | P | |||||||||||||||
N | P | P | P | P | P | P | P | P | P | |||||||
R | P | R | N | B | K | Q | B | N | R |
BOARD(10)
This game is played between four persons each one has an 8x8 chessboard.
9) For k=9, we have the (2x9+1) x (2x9+1) chess game, or the 19 x 19 chess game. Two players oppose each other. One has white pieces, the other has black pieces. The initial arrangement of pieces(pawns) is as in board(11). Each player has 2 Rooks, 8 knights, 8 Bishops, 1 King, and 19 pawns. The pieces move and capture as those of the 17 x17 chess game. The Knight can move 4 squares vertically and three squares horizontally and vice-versa. At the start of the game, the pawn has the option of moving 6 squares at a time, then he can move, one or two squares at a time. The prise en passant is still valid. The King can move one, two or three squares at a time. The castling is as follows:
Small castling: The King on j1 moves to p1, and the Rook on s1 moves to o1.
Great castling: The king on j1 moves to d1 and the Rook on a1 moves e1.
In this game, the lack of the Queen is compensated by the presence of 8 knights and 8 bishops on both sides of the King. This game can be also played as a four handed 9x9 chess game.
R | N | N | N | N | B | B | B | B | K | B | B | B | B | N | N | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | N | N | B | B | B | B | K | B | B | B | B | N | N | N | N | R |
BOARD(11)
We can continue the sequence of (2k+1) x (2k+1) chess games, for k a positive integer.
In this article I will limit the sequence to the 19 x19 chess game which is still playable and cannot take longer than the 8x8 chess game if we use the extended moves of the pieces. What we observe in the sequence of (2k+1) x (2k+1) chess games is that the King is always the point of symmetry and in both sides of it are specific number of Knights, and Bishops for specific positive integers k.
Mathematical Remarks
The following table(1) gives the number of Knights, Bishops, Queens and pawns used in the sequence of (2k+1)x(2k+1) chess games:Now, for each positive integer k, there are two Rooks, k pawns and 1 king for the player( with white or black pieces). From the above table(1) we can deduce that for the sequence of (2k +1)x (2k +1) chess games, k a positive integer, the number of Knights, resp. Bishops and Queens used are given by:
X = ( 2s Bishops, resp Knights and 0 Queen) if k is odd, greater than 2, and s is a positive integer.
Y = (2m Bishops, resp Knights and 2 Queens) if k is even greater than 2, and m is a positive integer
The castling follows the equality:
Small Castling: King moves 2q squares, q is a positive integer, and the Rook moves 2 squares.
Great Castling: King moves 1 square and the Rook moves 2d squares, d is a positive integer.
k Chess # BISHOPS # Knights #Queens # PAWNS =================================================================== 1 3x3 0 0 0 3 =================================================================== 2 5x5 0 1 0 5 =================================================================== 3 7x7 2 2 0 7 =================================================================== 4 9x9 2 2 2 9 =================================================================== 5 11x11 4 4 0 11 =================================================================== 6 13x13 4 4 2 13 =================================================================== 7 15x15 6 6 0 15 =================================================================== 8 17x17 6 6 2 17 =================================================================== 9 19x19 8 8 0 19 =================================================================== 10 21x21 8 8 2 21 =================================================================== 11 23x23 10 10 0 23 =================================================================== 12 25x25 10 10 2 25 =================================================================== 13 27x27 12 12 0 27 =================================================================== 14 29x29 12 12 2 29 =================================================================== 15 31x31 14 14 0 31 =================================================================== 16 33x33 14 14 2 33 =================================================================== 17 35x35 16 16 0 35 =================================================================== 18 37x37 16 16 2 37 ================================================================== 19 39x39 18 18 0 39 ================================================================== 21 41x41 18 18 2 41 =================================================================== etc. .............................................................. ...............................................................
Now, for each positive integer k, there are two Rooks, k pawns and 1 king for the player( with white or black pieces). From the above table(1) we can deduce that for the sequence of (2k+1) x (2k+1) chess games, k a positive integer, the number of Knights, resp Bishops and Queens used are given by:
X =( 2s Bishops, resp Knights and 0 Queen) if k is Odd and greater than 2, and s is a positive integer
Y = (2m Bishops, resp Knights and 2 Queens) if k is even and greater than 2, and m is a positive integer.
The castling follows the equality:
Small Castling: King moves 2q squares, q is a positive integer, and the Rook moves 2 squares.
Great Castling: King moves 1 square and the Rook moves 2d squares, d is positive integer.
SEQUENCE OF 2k x 2k CHESS GAMES
By applying the same approach of the sequence of the (2k+1) x (2k+1) chess games to the 2k x 2k chess games, where k is a positive integer difeerent from the powers of two, we can derive the following chess games, some of which were already established (the 10x10 chess, 12x12 chess and the 14x14 chess games). However, the approach we use here is different and, I believe that the study of sequences of chess games ( 2k x 2k, (2k+1) x (2k+1) and 2k x 2k chess games) were not studied as a whole.
Now, let consider the sequence of 2k x 2k chess games:
For k=3, we have the 2x3 x 2x3 chess game, or the 6x6 chess game. The initial arrangement of pieces(pawns) is as follows:
P | N | F | K | P | N |
P | N | F | K | N | P |
BOARD(1)
In this chess game, each player has 2 pawns, 2 Knights, 1 Firzan and 1 King.
The pawn moves and captures as the pawn of the 8x8 chess. The prise en passant is valid.
When promoted, it can be a knight, a firzan, or a pawn. The knight moves 1 square vertically and 1 square horizontally and vice-versa. The Firzan moves and captures one square diagonally.
The King moves and captures like the King of the 8x8 chess. There
is no castling.
For k=5, we have the 2x5 x 2x5 chess game, or the 10 x 10 chess game. The initial arrangement of pieces pawns is as in the board(2). Each player has 2 Rooks, 4 knights, 2 Bishops, 1 King and 1 Queen and 10 pawns. At the start of the game, the Rook, Queen, Bishop and King move and capture like those of the 8x8 chess game. At the start of the game, the pawn can moves one, two or three squares at a time. The castlings are simply like the castling of the 8x8 chess game.
R | N | N | B | Q | K | B | N | N | R |
P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P |
R | N | N | B | Q | K | B | N | N | R |
BOARD(2)
For k=6, we have the 2x6 x 2x6 chess game, or the 12 x 12 chess game. The initial arrangement is as in board(3). Each player has 2 Rooks, 4 Knights, 4 Bishops, 1 Queen, 1 King and 12 pawns. The Rook, Bishop, Knight, Queen and King move and capture like those of the 8x8 chess game. However, the King has the option of moving one or two squares at a time.
At the start of the game, the Pawn has the option of moving one, two, three, four or 5 squares at a time. The prise en passant is valid. When promoted it can be any of the pieces or a pawn, except for the King. The castlings is as follows:
Small castling: The King on g1 moves to k1, and the Rook on l1 moves to i1 .
Great castling: The King on g1 moves f1, and the Rook on a1 moves to g1.
R | N | N | B | B | Q | K | B | B | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | B | B | Q | K | B | B | N | N | R |
BOARD(3)
For k=7, we have the 2x7 x 2x7 chess games, or the 14 x14 chess games. The initial arrangement of pieces (pawns) is as in board(4). Each player has 2 Rooks, 6 Knights, 4 Bishops, 1 Queen, 1 King and 14 pawns. At the start of the game, the pawn has the option of moving one, two, three, four, five or six squares at a time. The Rook, Bishop, Queen move and capture like those of the 8x8 chess games. The King can move one, two or three squares at a time. The castling is as follows;
Small castling: King on h1 moves to l1, and Rook on n1 moves to k1
Great castling: King on h1 moves to g1 and the Rook on a1 moves to h1.
R | N | N | N | B | B | Q | K | B | B | N | N | N | R |
P | P | P | P | P | P | P | P | P | P | P | P | P | P |
P | P | P | P | P | P | P | P | P | P | P | P | P | P |
R | N | N | N | B | B | Q | K | B | B | N | N | N | R |
BOARD(4)
For k=9, we have the 2x9 x 2x9 chess game, or the 18 x18 chess game. Without drawing the 18 x18 chess board we can deduce from the preceding 2k x2k chess boards, that each player will have 2 Rooks, 6 Knights, 6 Bishops, 1 Queen, 1 King and 18 pawns.
Etc...
Probably, I will leave it to the reader to devise the following 2k x 2k chess games for k greater than 9.
Written by: A. Missoum. Edited by Hans Bodlaender; graphics for first five boards by David Howe.
WWW page created: April 2, 1997. Last modified: January 12, 1998.
The html-file was obtained from a document in Wordperfect, which was first converted to Word, and then from Word to html. Both conversions may have introduced errors, apologies for this. Also, Microsoft Explorer might display the tables in a wrong way - Netscape Explorer seems not to have this problem.
