Towards the theory of Byzantine Chess
The rules of
Byzantine Chess are
known but its theory did not survive.
An attempt of its reconstruction is given on this page.
The material is selected in the following topics:
- The
pieces on circular board
- The
motivation of playing on the circular board
- Strategy
- The
quick mates
- Symmetric
version of Byzantine chess
- The
relative values of pieces
1.The orthodox chess pieces on
the square and circular board
2.The shatranj pieces on various
boards
3.Comparison between the Byzantine
Chess and Circular Chess
The
Byzantine Chess endgames will be discussed on a separate page.
For other
information see the LINKS to
Byzantine Chess.
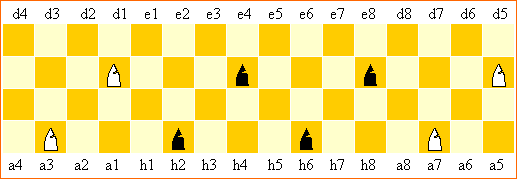
The
pieces on circular board
Notation on the circular
board
Byzantine Chess is a
variant of Shatranj
played on the round board (with perimeter of 16 and width of 4 squares).
This board
can be obtained from the standard 8x8 board separated into two halves:
the first including the Q-side (files a-b-c-d) and the second including
the K-side (files e-f-g-h).
Then, this two strips are glued so that their 1st and 8th ranks are connected.
(namely, a1-b1-c1-d1 with e1-f1-g1-h1 and a8-b8-c8-d8 with e8-f8-g8-h8).
Thus, the closed circular
board is obtained.
It is convenient to imagine it in the rectangular diagram
as in the Java and Zillions-of-Games
computer programs playing Byzantine Chess.
One should merely bear in mind that the left and right edges are glued,
e.g. a King from d4 may go on d5 and c5.
We do not know how the
Constantinople chessplayers denoted the squares,
but the above notation used in Circular
Chess
(the modern version of Byzantine Chess) looks natural and convenient.
The centre and the edges
The files a
and h are combined into the single string which now has the form of
closed ring.
Starting from the arbitrary point on ring a-h and going along it, a King
will return to the initial position having passed the closed circle of 16
squares.
The rest three files (rings) are d-e, b-g and c-f.
The rings b-g
and c-f constitute the center of the board,
while its edges are formed by the rings a-h and d-e.
Thus, there are only two different types of squares:
the full space is divided in equals halves between
the 32 central squares and 32 bordering squares.
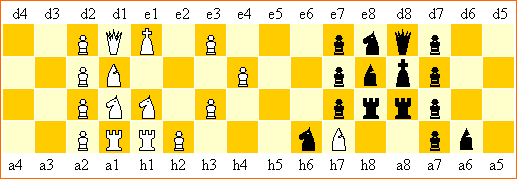
In the
frames of the accepted notation the
pieces in the opening stand at the same positions as denoted for them in the
orthodox chess.
For example, white Rooks stand on a1 and h1 etc.
Therefore, the initial setup in rectangular development looks as
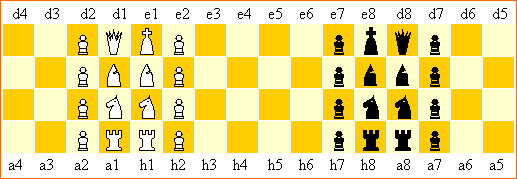
On the relevant circular
development the kings stand on the inner perimeter
and the CW rotation on the circular board corresponds to the motion from right
to left on the rectangular development.
The opposite direction
(left to right) corresponds to the CCW rotation.
Therefore the CW or left
Pawns are:
a2, b2, c2, d2 (White)
and
e7, f7, g7, h7 (Black).
The CCW or right Pawns are:
e2, f2, g2, h2 (White)
and
a7, b7, c7, d7 (Black).
Each
partner has two separate arrays of pawns (four pawns at the K-side and four
pawns at the Q-side).
The pawns
have different significance depending on their position.
There are only two principle positions for Pawns: in the centre (more valuable)
and at the edge.
In the initial setup either player has four pawns of both kinds.
Pawns at the edge
The Rook's
Pawns at K-side and Q-side are very similar to each other because either
has two defenders (a Rooks and a Knight).
But the Queen's
Pawn, supported only by the King's Knight, is weaker than the King's
Pawn, protected twice.
Central Pawns
The Bishop's
Pawns are also twice protected (c-Pawn is supported by Queen, f-Pawn is
supported by King and both - by Knights).
The weak Knight's
Pawns are not protected at all.
But the place of the Knight's Pawn at the K-side (g2 and g7) must be
considered as the weakest point, because the enemy's Knight, appeared on it,
checkmates the King as it happens in the scholar's
mate.
Whereas the
pieces obey the shatranj
rules (with few exceptions
concerning the pawns), the geometry of circular board adds new properties.
Namely
- The Kingside and Queenside are separated.
- The Pawns may go either clockwise or
counterclockwise.
- The strength of Rooks is increased.
These
reasons are enough to explain the choice of the circular board.
However, the most striking difference from Shatranj is not so evident because
it concerns the transformation of the Bishops' trajectories on the round board.
In fact, the Bishops of Byzantine Chess are endowed with quite new
possibilities.
According to the shatranj
rules, a Bishop jumps diagonally over one square so that his move is a slip in
two diagonals.
On the square board the Bishops of the opponents never collide.
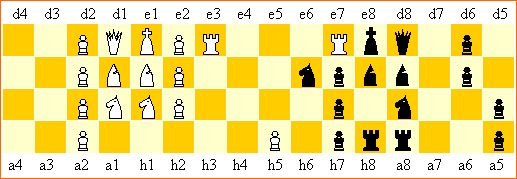
Diagram
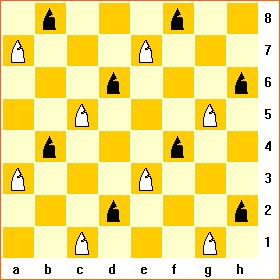
depicts the
full set of squares available for the dark-coloured Bishops.
The dark-coloured Bishop of White has the initial stay at c1 and cannot
reach f8 where the dark-coloured Bishop of Black sits in the opening.
The paths of Bc1 and Bc8 have no point of intersection.
The light-coloured Bishops
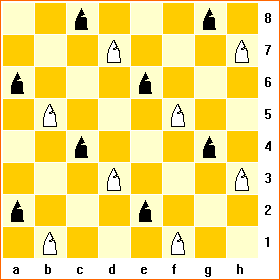
also never meet.
Therefore, all four Bishops
keep neutrality.
The circular board of
the Byzantine Chess provides interaction of the Bishops and
peaceful co-existence is now impossible.
Each Bishop goes along the
strictly determined trace.
Among the eight squares available to him: four are on the central c-f
file and four are on the bordering a-h file.
The dark-coloured Bishop can stay on c1, c5, f8, f4
and a3, a7, h6, h2
while the light-coloured Bishop can stay on f1, f5,
c8, c4 and h3, h7, a6, a2
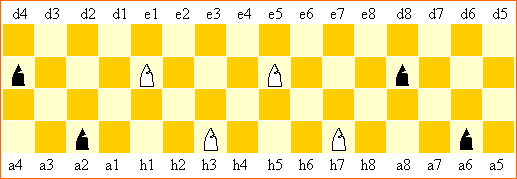
The Bishops of identical
colour go the same path and can capture each other.
The rule of moves have not
been changed but the Bishops reveal sufficiently new behavior.
How could it happen?
On the square board a
Bishop from the K-side is transferred to the Q-side passing the
"equator" between the files d and e.
On the circular board the direct communication between the K-side and Q-side
does not occur.
Hence, if a piece goes from the K-side to the Q-side, his short route, drawn on
the square board, transforms in a long march on the round board (and
conversely).
The "interests"
of Bishops from the opposite armies clash on the squares of common colour and
this fact forms the background for new tactical motives in Byzantine Chess.
Thus, playing on the circular board is not a peculiar fantasy of Byzantine
scholars.
Strategy: the Kingside or
Queenside?
It is seen
from the initial setup that the
circular board is divided into two distinct parts, because
the K-side (files e-f-g-h) and the Q-side (files a-b-c-d) are disconnected and
can be called more properly as fronts rather than wings.
Contrary to the chess on 8x8 board, the Queen's and King's sides live their
individual life
and advance at one side cannot be counterbalanced at the other side.
As a rule the main events take place at the K-side.
The
attack at the Q-side is not so prospective as on the square board.
- The Q-side game was reasonable in view of
making a breakthrough and the passed Pawns.
- This policy was possible due to the
pressure of figures.
- The Q-side development usually involves
activity in the centre.
On the round board these factors are absent.
- The Pawns have promotion and cannot become
a serious threat.
- The weak Bishop and, especially, Queen can
contribute very small to destruction of the opponent's defense.
- The circular board has no centre in the
sense of four squares d4-e4-e5-d5 and there is no possibility to
contribute simultaneously from the K-side because it is separated from the
Q-side.
Thus, the
early development of the Q-side is dubious, because the opponent can rapidly
mobilize his forces at the K-side for a sharp and decisive attack.
The strong Queen and Bishop of Circular
Chess provides theoretical possibility for the Q-side
attack, although the main events take place at the K-side very often,
particularly in the central game of the recent Circular
Chess championship.
The quick mates
In
Byzantine Chess the quickest "scholar's" checkmate is accomplished by
a Knight in four moves.
White do it so:
1. Ng1-h3 a7-a6
2. Nh3-f4 a6-a5
3. Nf4- h5 Ra8-a6
4. Nh5 x g7#
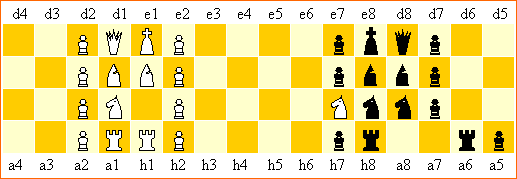
Another variant:
1. Ng1-f3 -- 2. Nh4 -- 3. Nf5
-- 4. Nf5 x g7#
with the same finale.
In the similar manner and
also at the fourth move such checkmate can be declared by Black.
Namely,
1. -- Ng8-h6 2. -- Nh6-f5 3. -- Nf5-h4 4.
-- Nh4 x g2#
or
1. -- Ng8-f6 2. -- Nf6-h5 3. -- Nh5-f4 4.
-- Nf4 x g2#
There are many ways to
checkmate by a Knight in 5 and 6 moves.
A Bishop can also
declare a smothered checkmate, however, not sooner than at the 7th
move.
For example:
1. Bf1 - h3 Bc8 - a6
2. g2 - g3 Ke8 - c8
3. f2 - f3 Nb8 - e8
4. f3 - f4 Ra8 - b8
5. e2 - e3 Ng8 - h6
6. Bh3 - f5 Rh8 - g8
7. Bf5 x h7 #
A
mate by Pawn also requires, at least, 7 moves:
1. e2-e3 -- 2. e4 -- 3. e5 -- 4.
Ng1-f3 -- 5. Nf3-g5 -- 6. e6 -- 7. e6 x
f7#

A Rook
checkmates not sooner than at the 8th move.
For example
1. h2 - h3 Ng8-f6
2. h3 - h4 B f8 - h6
3. h4 - h5 b7 - b6
4. Rh1 - h4 Bh6 - f8
5. Ra1 - h3 Bf8 - a7
6. Rh4 - e4. Ba7 - f8
7. Rh3 - e3. a7-a6
8. Re4 x e7#
A Queen (who goes only one
diagonal) is able to prepare a checkmate at the 11th move:
1 move by a Pawn (e or g) plus
1 move by the Bishop f1 (in order to open the way out for
the Queen),
8 moves for the Queen to reach f7;
2 moves for the Knight g1 to land on e5 or h6 (in
order to support the Queen):
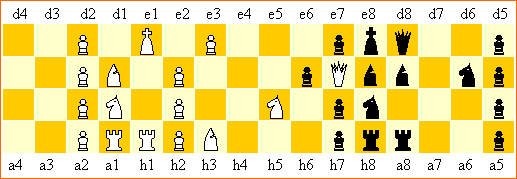
In this alternative version
of Byzantine chess
the initial
positions of the black King and Queen are replaced so that
the black King is situated on the dark square while the black Queen stands on
the light square:
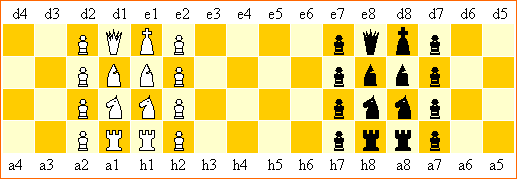
Such replacement results in
the appearance of interaction between the Queens.
A Queen steps one square diagonally and may go on the squares of one colour as
a Bishop of modern chess.
In the ordinary Byzantine Chess the Queens never intersect, because the white
Queen goes on the light squares, while the black Queen goes on the dark
squares.
In the symmetric Byzantine Chess both Queens may walk on the same squares
(light on the above diagram).
But the main difference
from the ordinary Byzantine Chess concerns the strategy rather than tactics.
Now the Kingsides and
Queensides are situated symmetrically (as in modern chess after castling on the
opposite sides). .
As a result the game develops at both fronts.
From his Q-side White attacks the K-side of the opponent,
while Black has better chances at his Q-side situated in front of the K-side of
White.
The relative values of
pieces
In order to
determine the game principles it is important to specify the prices of pieces.
Since the pieces of Byzantine Chess obey the shatranj
rules
the strongest figure is Rook, the middle is Knight, and the two weakest are
Queen and Bishop (they have approximately equivalent power).
So, there are three classes of figures and the Pawns.
The properties of
shatranj pieces are modified on the circular board (for example, right
defense in the endgame K+R vs K allows to avoid a mate).
The most natural way to get precise theoretical estimations is practice,
particularly
the Zillions-of-Games
or Java
computer programs may allow to acquire some game experience.
Thus,
for the first step we have a plain arithmetical definition of the pieces
strength as an average number of squares being under its control.
A Knight
controls:
9 squares when it stands in
the centre of the 8x8 board (it goes on 8 squares plus the square on which it
stands),
and only 3 when it stands in the corner.
There are no corners on the circular board, while the number of central squares
and the squares at the edges is the same (32).
A Knight on the circular board controls:
7 squares when it stands in the centre:
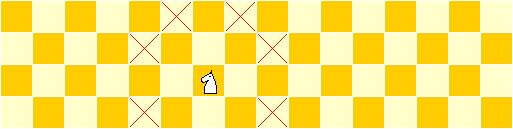
and 5 when
it stands at the edge:
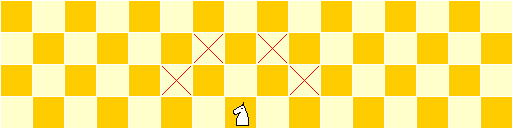
Hence, the average value of Knight on the circular board is (7 x
32 + 5 x 32) / 64 = 6.
All 64 squares are available
for Rook and it controls 19 squares without regard of its
situation:
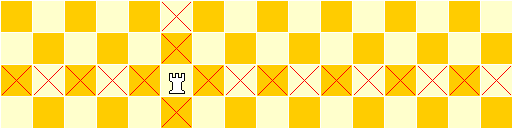
the whole ring of 16 squares (including the square on which the Rooks
stands) and 3 squares in the transversal direction.
(Rook on the 8x8 board controls 15 squares simultaneously).
Queen and Bishop
Queen and Bishop form the
class of weak figures whose significance twice smaller than 1 Knight
and corresponds to 1.5-2 Pawns.
A Queen can
stand on each of 32 squares corresponding to its color.
Being in centre, it controls 5 squares,

and only 3 -- when it stands at the edges
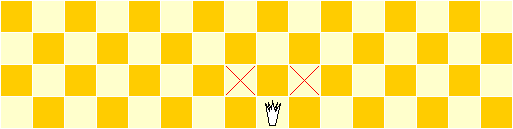
Therefore, the average is (5 x 16 + 3 x
16) / 32 = 4.
In fact, a Queen of orthodox chess is much stronger: on the circular board it
holds 27 squares simultaneously.
The
Byzantine Chess Bishop
controls 3 squares without regard of its situation.
A Bishop of orthodox chess includes the functions of shatranj Queen and Bishop.
On the circular board he always controls 7 squares.

A Pawn holds 2
squares: the first on which it stands and the second on which it can land.
This evaluation corresponds to the free board where the capture is not
included.
However, it does not increases the Pawn strength in the real game because
the opponent's Pawns or figures can often block the motion.
The estimation of 2 implies that a Pawn is three times weaker
than a Knight (6).
The evaluation (9 x
32 + 6 x 32) / 64 = 7.5 derived for a King is not so
informative,
because this piece stands aside from other pieces for he cannot be captured or
exchanged.
The averaging over square
board is slightly more complicated and the similar calculation can be done for
arbitrary board and set of pieces.
It is convenient to express
the strength of pieces in the pawn equivalent (1 unit for a Pawn).
The orthodox chess pieces
on the square and circular board
The
relative strength of orthodox chess pieces is selected in the table below:
|
||
|
Square board |
Circular board |
|
Rook |
7.5 |
9.5 |
|
Knight |
3.06 |
3 |
|
Queen |
12.38 |
13.5 |
|
Bishop |
5.06 |
3.5 |
|
King |
3.78 |
3.75 |
The strength of long-range
figures (Queen, Rook, Bishop) corresponds to the free board and is always overestimated,
because in real games they often act in the closed positions.
Nevertheless, it is clear that such figures become much stronger on the
circular board, while the other pieces remain at approximately their ordinary
level.
It is strange that on the
round board a Knight has become even weaker.
The corners of the square board are compensated by the absence of
"deep" centre inside the round board: indeed, this circular strip it
is very narrow (only 4 cells), or better to say, "shallow", for a Knight.
Only on the torus shaped board without edges the Knight achieves his maximal
strength.
In order to get the
realistic values for the long-range figures, approximately two units must be
extracted from the above numbers.
Hence,
Queen = 10
Rook = 5.5
Bishop = 3
on the square board, while
Queen = 11.5
Rook = 7.5
on the
circular board.
A Bishop on the narrow
circular board does not belong to this class, because its trace does not exceed 4
squares.
Therefore the estimation of Bishop must be reduced very little so that its
price will not be much higher than that of a Knight.
The shatranj pieces on
various boards
The
estimation of the shatranj pieces looks so:
|
|||
|
Shatranj |
Byzantine Chess |
Toroidal Byzantine Chess |
|
Rook |
5.5 (7.5) |
7.5 (9.5) |
7.5 (9.5) |
|
Knight |
3.06 |
3 |
4.5 |
|
Queen |
2.03 |
2 |
2.5 |
|
Bishop |
1.5 |
1.5 |
1.5 |
|
King |
3.78 |
3.75 |
4.5 |
The values without correction
(for the long-range moves) are given in brackets.
A Rook has become stronger
than a pair of Knights,
and the standard formula Rook + Pawn = 2Knights
requires revision.
Comparison between the
Byzantine Chess and Circular Chess
The summary
strength of the Circular Chess pieces is 53.25 (with correction).
The relevant value for the
Byzantine Chess is only 37.75.
These numeric estimations
reflect the tactical contents of both chess variants.
The weak Queens and Bishops of Byzantine Chess result to the evident difference
from the Circular Chess:
the complex combinations in Byzantine Chess appear rare and strategy often
prevails over tactics.
As a result, the most common and trivial method to win is the utter capture of
the opponent's forces
(bare king is recognized as loss), while checkmate (and stalemate, declared
also as win) takes place relatively rare.
Exchanges
The
knowledge of the pieces' values is necessary for making correct exchanges.
On the
square board 2 Knights are nominally better than 1 Rook.
On the circular board the exchange of 2N for 1R
is quite well.
In fact, the Rook on the circular board is almost 1.5 times
stronger than it was on the square board.
As a result, the formula
R = 2N + B
or
2N + Q > R > 2N+1P
suits to this exchange.
The table of the pieces values allows to write the
other expressions
N = 2B = Q + P
Q > B > P
The exchanges
Q = 2P
B < 2P
Q < B + P
are less evident, because they strongly depend on the particular game
background and the form of the Pawns array.
GO TO THE TOP OF
THE PAGE
OTHER LINKS TO BYZANTINE CHESS
Written by Anatoly Khalfine and Ernst Saperow
To contact the authors, please email: (email removed contact us for address) os.com
WWW page created: August 19,
2002.