Small Spherical Chess
Introduction
Early in November, we received the following email from Andrea Mori regarding a variant he had invented for the 38-challenge contest:I attach my entry for your Contest for a board with 38 squares.
Actually, I cannot swear that my construction is fully original, so feel free to reject the entry if you can find it elsewhere.
Best wishes!
Andrea Mori
The Board
Let us start with a description of a model for a spherical board. I already described this model in Eteroscacco. [Eteroscacco Magazine is the bulletin of AISE, an Italian organization playing chess variants. Highly recommended, provided that you are able to read Italian!]
A sphere can be constructed as follows. Take a square, glue two opposite edges to form a cylinder. Then close the cylinder by gluing a disc to the top edge and a disc to the bottom edge. The resulting surface is a sphere.
So, let us start with an n x n chessboard and first glue the first column to the last column. This is the classical cylindrical chessboard of order n. Then add two squares: a square called N (North Pole) and a square called S (South Pole). These two squares are actually n-gons: the n edges of N (resp. of S) are glued to the top edges of the squares in the n-th rank (resp. the bottom edges of the squares in the first rank). Thus N is "above" each square in the top rank, and S is "below" each square in the bottom rank.
This spherical chessboard consists of n2+2 squares.
If we slice a sphere with a plane containing both poles, we obtain a circle consisting of the union of two opposite meridians. On our spherical chessboard, the meridians are the columns, and two columns are "opposite" (in the sense explained above) if their distance is n/2. To make this well-defined, we shall assume that n is even.
Movement
We have to discuss separately movement from (or to) a pole and movement through a pole. To fix ideas, we shall refer to the N square and take n=8. The discussion for S and other values of n is completely analogous.
To define the movement of pieces from N, it is convenient to think of N as "x9" where x is a generic column. Thus, on an empty board:
- a Rook can move from N to any square on the board by moving along a column;
- a Bishop in N can reach any square on the board in two distinct ways (for instance a B can reach b6 either via d8-c7-b6 or h8-a7-b6, remember that the board is cylindrical);
- a Knight in N can move to any square in the 8th or 7th rank;
- a King in N can move to any square in the 8th rank;
- a white Pawn anywhere in the 8th rank can move to N or capture a Black piece in N (i.e. pawns in their 8th rank are unblockable). White pawns are promoted when they reach N.
The movement through N concerns only long-range pieces:
- a Rook enters N by moving along a column a may leave it by moving along the opposite column (see previous discussion). E.g. a Rook in b6 can move to f7 via b6-b7-b8-N-f8-f7.
- a Bishop moves along oblique lines that make constant angles with the columns. Think of N as reduced to a single point and each 8th rank square joined to its opposite by their topo edges. The Bishop's movement follows. For instance, a Be7 can move e7-f8-N-c8-d7-... or e7-d8-N-g8-f7-....
A Board With 38 Squares
If we take n=6, the resulting spherical chessboard has 62+2=38 squares!
It only remains to define a starting position.
Given the smaller size of the board, let's get rid of Queens and start with:
White: K in S, Ra1, Bb1, Nc1, Nd1, Be1, Rf1, Pawns a2 to f2.
Black: K in N, Ra6, Bb6, Nc6, Nd6, Be6, Rf6, Pawns a6 to f6.
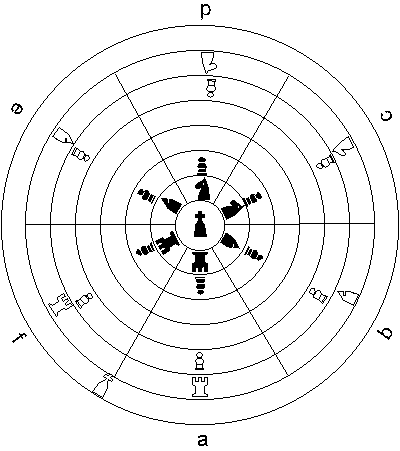
[Editor's note: please forgive the rather crude diagram. However, it does give a convenient, if distorted, view of the "board". We have simply taken the sphere, punched a hole in the southern pole, and then pulled the sphere out flat, so that the outermost ring is what had been the southern pole. -D.H.]
Finally, there is no castling and no initial two-steps pawn moving (and so, no en-passant either).
Written by Andrea Mori. Diagram and HTML conversion by David Howe.
This is an entry in the Contest to make a chess variant on a board with 38 squares.
WWW page created: November 8, 1997. Last modified: November 14, 1997.
